《固体物理学》课程教学课件(PPT讲稿)Chapter 7 The electronic theory of metal(supplement - Sommerfield expansion)

7-1 supplement:Sommerfield expansion Set O(E)is continuously diffrentiated between (-co,+o), (0)=0,and lime afEy≥IfkeT<Er, prove that 1-r(E)0'(e)证=Q(e,)+石k,7Q'(E,) where f(E)=- F-D function E-EE
7-1 supplement: Sommerfield expansion Set Q(E) is continuously diffrentiated between(-,+), Q(0)=0 ,and ,α>0。If kBT << EF, prove that lim 0 ( ) E E e Q E − → = ( ) ( ) ( ) ( ) ( ) 2 2 0 d 6 F B F I f E Q E E Q E k T Q E = + where ( ) 1 exp 1 F B f E E E k T = − + F-D function
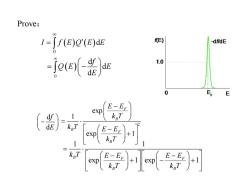
Prove: I=Jf(E)0(E)dE f(E) -dfdE -j()r 1.0 0 E E E-Eg exp kgT kgT exp E-EE +1 kgT kgT
Prove: ( ) ( ) 0 I f E Q E Ed = ( ) 0 d d d f Q E E E = − d d f E − 2 exp 1 exp 1 F B B F B E E k T k T E E k T − = − + 1 1 exp 1 exp 1 B F F B B k T E E E E k T k T = − − + − +

We find that (-df7dE)is a even function of (E-E), the value of (-dfdE)is mainly focused nearby the scope of E-EF~kBT,if E-EFl>n kBT,the value of the function is approaching 0 rapidly,similar to the function of δ So,the contribution to the integral is mainly focused in the small scope of E~Er,because Er>>kBT,the lower limit of the integral is replaced by -oo from 0,and the integral value is supposed not to be changed. 1=joae
(-df/dE) is a even function of(E-EF), the value of (-df/dE) is mainly focused nearby the scope of E-EF kBT,if E-EF > n kBT,the value of the function is approaching 0 rapidly, similar to the function of δ So, the contribution to the integral is mainly focused in the small scope of E ~ EF,because EF >> kBT,the lower limit of the integral is replaced by -∞ from 0,and the integral value is supposed not to be changed. ( ) d d d f I Q E E E − = − We find that

perform the Taylor expansion on O(E)nearby E=EE (E)=(E)+E)(E-E)+"(Er)(E-E)'+. =iee,〔}r+jQ(Ee-,〔ur +5Q(,6-R,{)c+ wherej()()
perform the Taylor expansion on Q(E) nearby E=EF ( ) ( ) ( )( ) ( )( ) 1 2 2! Q E Q E Q E E E Q E E E F F F F F = + − + − + ( ) ( )( ) d d d d d d F F F f f I Q E E Q E E E E E E − − = − + − − ( )( ) 2 d 1 d 2 d F F f Q E E E E E − + − − + ( ) ( ) 0 d d d F F f I Q E E Q E E − = − = where
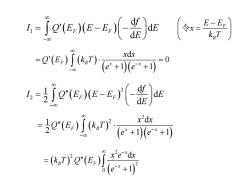
4-eec-¥s〔尝 -eie德可0 4=fQe,E-,() g7可 xdx -i
( )( ) 1 d d d F F f I Q E E E E E − = − − ( ) ( ) ( )( ) d 0 1 1 F B x x x x Q E k T e e − − = = + + F B E E x k T − = 令 ( )( ) 2 2 d 1 d 2 d F F f I Q E E E E E − = − − ( ) ( ) ( )( ) 2 2 d 1 2 1 1 F B x x x x Q E k T e e − − = + + ( ) ( ) ( ) 2 2 2 0 d 1 x B F x x e x k T Q E e − − = +

Perform Taylor expansion 0-1(,on-e,-g 3 -旷ga,订r02a4ja -eryos+s+ -T(k,Q(E) 1,11 1-+3 π2 12 [f(E)0'(E)dEQ(E,)+石(,T)'Q(E,)
( ) ( ) ( )( 1 1 2 ) 2 3 ( )( )( ) 1 1 2! 3! n n n n n n n − − − − − − − − − + = + − + + + Perform Taylor expansion ( ) ( ) 2 2 2 3 2 0 1 2 3 4 x x x x B F I k T Q E x e e e e dx − − − − = − + − + ( ) ( ) 2 2 2 2 1 1 1 2! 1 2 3 4 B F k T Q E = − + − + ( ) ( ) 2 2 6 B F k T Q E = 2 2 2 2 1 1 1 1 2 3 4 12 − + − + = ( ) ( ) ( ) ( ) ( ) 2 2 0 d 6 F B F f E Q E E Q E k T Q E +

7-2 supplement:the calculation of systematical energy at T>0 (EE-CI E2 dE rw-e y'dy F;(o)I(j+1)exp(o) Rw- %>1
3 2 0 0 ( ) ( )d d 1 exp F B E U EN E f E E C E E E k T = = − + 0 0 0 0 0 1 2 0 0 2 0 d ( ) 1+exp(y-y ) ( ) ( 1)exp( ) ( 1) ( ) 1 ( 1) 6 j j j j j y y F y F y j y y j j F y j y + = + + + + + 0 ( ) 2 − y 0 y 1 7-2 supplement: the calculation of systematical energy at T>0

3 5 E2 2 Er>>kgT 1+exp E-Ee 门 +会j] .j T<<Eg/kg
3 5 2 2 2 0 2 5 d 1 - 5 8 1+exp F B F F B E E k T E E E E k T + E k T F B 5 2 2 0 2 0 5 2 0 2 0 2( ) 5 5 1 1 5 24 8 2( ) 5 1 5 12 F B B F F F B F E k T k T E E E k T E − + + ( ) 5 3 0 2 2 2 2 2 0 2 2 2 0 0 2 5 1 5 12 4 F B F B F E m k T U E n k T U E + = + 0 / T E k F B

7-3 supplement:the DOS at Fermi surface 3 n 2 322 一k,=(3 2m .N(E)= 3 n 2 E
7-3 supplement: the DOS at Fermi surface ( ) 3 3 1 2 2 0 0 0 2 2 2 2 2 2 2 2 2 3 2 2 2 1 2 1 2 ( ) 2 2 2 1 1 3 2 3 2 F F F F F F F F m m k N E C E E m m m n n k k k k m = = = = = = 0 0 3 ( ) 2 F F n N E E = ( ) 1 2 3 3 F k n =
按次数下载不扣除下载券;
注册用户24小时内重复下载只扣除一次;
顺序:VIP每日次数-->可用次数-->下载券;
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 6 Electron motion in the crystal 6.4 The electron motion in a constant electric field 6.5 Band structure of conductor, insulator and semiconductor.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 6 Electron motion in the crystal 6(supplement - a more concise description of quasi-momentum).ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 6 Electron motion in the crystal 6.1 The quasi-classical description of Bloch electrons 6.2. Electron quasi-momentum 6.3 The accelerated velocity and effective mass.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 5 band theory 5.4 Tight Binding Approximation(TBA).ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 5 band theory 5.3 Nearly Free Electron 5.4 BZ and energy bands.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 5 band theory 5.5 The symmetry of bands.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 4 lattice dynamics and lattice capacity 4(supplement - Thermal Expansion of Glass).ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 4 lattice dynamics and lattice capacity 4.6 Anharmonic Effects 4.7 Equation of states for Lattice 4.8 Experimental methods for the determinations of lattice vibration spectroscopy.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 5 band theory 5.1 general background and approximations 5.2 Bloch’s Theorem.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 4 lattice dynamics and lattice capacity 4.5 Heat capacity of the lattice.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 4 lattice dynamics and lattice capacity 4.1 Normal Modes of Vibration 4.2 Density of States 4.3 Harmonic approximation and normal mode coordinates 4.4 phonon.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 3 Interatomic Bonding 3.1 General crystal binding 3.2 typical binding.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 3 Interatomic Bonding 3(Supplement - Rules of crystal binding).ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 3 Interatomic Bonding 3.3 The cohesive energy for ionic crystal.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 2 Wave Diffraction & the Reciprocal Lattice(2.1-2.5).ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 2 Wave Diffraction & the Reciprocal Lattice 2.6 X-Ray Diffraction Methods 2.7 Applications of XRD.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 2 Wave Diffraction & the Reciprocal Lattice(supplement - Review & Overview of X-Rays).ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 1 Crystal Structure 1.9 Elements of Symmetry 1.10 Space groups 1.11 7 crystal system and 14 Bravis Lattice.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 1 Crystal Structure(Supplement - microstructure and crystal system).ppt
- 《固体物理学》课程教学课件(PPT讲稿)Introduction.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 7 The electronic theory of metal 7.1 Fermi statistics and the heat capacity of electron 7.2 Work function and contact potential.ppt
- 《固体物理学》课程教学课件(PPT讲稿)Chapter 5 band theory 5.6 Density of states(DOS)and Fermi surface 5.7 the electrons in the crystal 5.8 the experimental results for DOS.ppt
- 《近代物理实验》课程教学大纲.doc
- 《近代物理实验》课程教学资源(PPT课件讲稿,共五部分).ppt
- 《工程光学》课程教学大纲 Engineering Optics.doc
- 安徽大学:《工程光学》课程授课教案(讲义,共十三章,授课教师:李桂华).doc
- 《工程光学》课程实验指导书(共六个实验).doc
- 《量子力学》课程教学课件(讲稿)Chapter 4 态和力学量的表象 The representation for the states and dynamical variable.pdf
- 《量子力学》课程教学课件(讲稿)Chapter 1 绪论 Quantum mechanism(量子力学的诞生).pdf
- 《量子力学》课程教学课件(讲稿)Chapter 2 波函数和薛定谔方程 The wave function and Schrödinger Equation.pdf
- 《量子力学》课程教学课件(讲稿)Chapter 3 量子力学中的力学量 The Dynamical variable in Quantum Mechanism.pdf
- 《量子力学》课程教学课件(讲稿)Chapter 6 散射 Scattering.pdf
- 《量子力学》课程教学课件(讲稿)Chapter 5 微扰理论近似方法 Approximation(本征值问题的似解).pdf
- 《量子力学》课程教学课件(讲稿)Chapter 7 自旋与角动量、全同粒子体系 Spin and undistinguished similar particles.pdf
- 《量子力学》课程教学资源(习题解答)第七章 自旋与角动量、全同粒子体系.doc
- 《量子力学》课程教学资源(习题解答)第三章 量子力学中的力学量.doc
- 《量子力学》课程教学资源(习题解答)第二章 波函数和薛定谔方程.doc
- 《量子力学》课程教学资源(习题解答)第五章 微扰理论.doc
- 《量子力学》课程教学资源(习题解答)第四章 态和力学量的表象.doc
- 《量子力学》课程考试大纲(自考).doc
