厦门大学:《结构化学 Structural Chemistry》课程教学资源(课件讲稿)Chapter 2 Atomic Structure

Chapter 2 Atomic Structure
Chapter 2 Atomic Structure
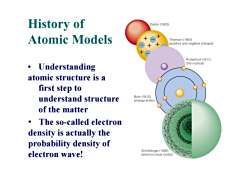
History of Dalton (1803) Atomic Models Thomson(1904) (positive and negative charges) Rutherford(1911) Understanding (the nucleus) 。 atomic structure is a first step to understand structure Bohr(1913) (energy levels) of the matter The so-called electron density is actually the probability density of Schrodinger(1926) electron wave! (electron cloud model)
History of Atomic Models • Understanding atomic structure is a first step to understand structure of the matter • The so-called electron density is actually the probability density of electron wave!

2.1 The Schrodinger equation and its solution for one-electron atoms 2.1.1 The Schrodinger equation The Hamiltonian Operator of one-electron atoms H atom,Het and Li2+ 月=i+元+。= 2mn V2+V-e e The reduced mass u= M·me 1836m。 ≈0.999m。 M+me (1+1836)m
2.1 The Schrödinger equation and its solution for one-electron atoms 2.1.1 The Schrödinger equation The Hamiltonian Operator of one-electron atoms H atom, He + and Li2+ e n e e n n n e n e V m m H T T V ˆ 2 2 ˆ ˆ ˆ ˆ 2 2 2 2 1836 0.999 (1 1836) e e e e e Mm m m Mm m The reduced mass e n

Consider that the electron approximately surrounds the atomic nucleus,the Hamilton operator can be simplified as 、 +n 2m。 = 方2 2 2m。x+ n-e Ze2 e n-e 4π8o' r=vx2+y2+22 The Schrodinger equation Hy =Ew Separation of variables
e n e e e n e V m H T V ˆ 2 ˆ ˆ ˆ 2 2 r Ze Vn e 0 2 4 ˆ Consider that the electron approximately surrounds the atomic nucleus, the Hamilton operator can be simplified as r e 2 2 2 r x y z 2 n-e 2 2 2 2 2 e 2 V ˆ ) x y z ( 2m H - ˆ Separation of variables ? The Schrödinger equation Hˆ E
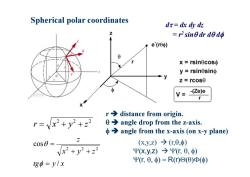
Spherical polar coordinates dr-dx dy dz Z r2 sin0dr dedo e(reo) X=rsinθcoso y=rsinθsino z=rcosθ V= -(Ze)e X r>distance from origin. r=vx2+y2+22 0>angle drop from the z-axis. φ→angle from the x-axis(onx-y plane) c0s0= (xy,z)→(c,0,φ) Vx2+y2+22 Ψ(X,y,Z)→ΨC,0,) tgo=y/x Ψ,0,φ)=R(0)⊙(0)Φ(φ)
Spherical polar coordinates 2 2 2 r x y z (x,y,z) (r, ) x,y,z r, r, R r r distance from origin. angle drop from the z-axis. angle from the x-axis (on x-y plane) 2 2 2 cos x y z z tg y / x d = dx dy dz = r2 sin dr d d
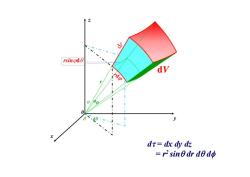
dr rsingd dV rdo p dr=dx dy dz =r2sinθdr de do
d = dx dy dz = r2 sin dr d d

Partial derivatives x=rsinecoso r=vx2+y2+22 y=r sinesing z=rcos0 c0S0= Vx2+y2+22 tgo=y/x +y2+22)i2x==sin0c0s0 ∂0 -sin0 Ox a0cosθcosp 8x
Partial derivatives 2 2 2 r x y z 2 2 2 cos x y z z tg y / x x = r sin cos y = r sin sin z = r cos ( ) 2 sin cos 21 21 2 2 2 rx x y z x xr x r r zx z x y z x x cos cos )( ) 2 21 sin ( 3 2 2 2 3/ 2

Spherical polar coordinates dx ax or dx 80 ox op a.cos0cosφo sino a =sin0cosφ Or 00 rsine do =sinosin 0+cos8sinp.0+cos中.0 3 00 rsine 0d a =cos0o sino o 0z Or r 60 72 10 1 1 r20 (sin 2 Or r2 sine 00 a0 r2sin20002 Ze2 n-e (4πE)r 1 1 1 r2 or ay+8πm(E+ Or o(sin+r sin2e 062 r2sine 80 a0 Ze'=0 4π6I
Spherical polar coordinates () ( ) ( ) r x xr x xp cos cos sin sin cos rr rsin cos sin cos sin sin y rr rsin z r r sin cos 2 2 2 2 2 2 2 2 sin 1 (sin ) sin 1 ( ) 1 r r r r r r 2 0 (4 ) n e Ze V r The Schrödinger equation 2 2 2 2 2 2 22 2 2 0 11 1 8 ( ) (sin ) ( ) 0 sin sin 4 m e Ze r E rr r r r h r

2.1.2 The solution---separation of variables SubstituteΨc,0,Φ)=R(ro(o)Φ(φ)into the equation 1 ay8πm(E+ r2 or r2sine 00 00 r2sin20062 h2 Zew=0 4π8' 2 and multiply with R(r)⊙(0)Φ() 1 oR) 1 + oΦ(@8元m(E+ R(r)or or ⊙(0)sin0a (sin) a0Φ()sin20ap2 h2 er2=0 π' 1 (r2R( 8mle(E+4π ze2 )r2=[ 1 (sin 1 a2Φ(φ) )+ R(r)ar (e)sine a0 ae Φ(p)sin20ap2
2.1.2 The solution --- separation of variables Substitute r, R r into the equation 2 2 2 2 2 2 22 2 2 0 11 1 8 ( ) (sin ) ( ) 0 sin sin 4 m e Ze r E rr r r r h r and multiply with ( ) ( ) ( ) 2 R r r ) 0 4 ( ( ) 8 ( )sin 1 ) ( ) (sin ( )sin 1 ) ( ) ( ( ) 1 2 0 2 2 2 2 2 2 2 r r ze E h m r R r r R r r e ] ( ) ( )sin 1 ) ( ) (sin ( )sin 1 ) [ 4 ( 8 ) ( ) ( ( ) 1 2 2 2 2 0 2 2 2 2 r r ze E h m r R r r R r r e

1 (rR((E (sin) 1 a2Φ(中) R(r)ar Or h2 4π6' (e)sine a0 a0 Φ()sin20ap2 1 let ((r) ( 8元2m2(E+ e )r2= R(r)or Or h2 4πEJ Radial part a (sin0 o0(8) a2Φ(p) + Angular part (0)sine a0 a0 Φ(p)sin20ap2 multiplied sin20 sine sin6o0②)+1 ⊙(0)a a01 ()=-Bsin20 ()62 sine o (sin) 80)+Bsin'0=- 1 aΦ(p) ⊙(0)a D()8d2 sine 6 let (sin+Bsinm (Θ ⊙(0)a a0 eq) 1 aΦ()=-m ()02
2 2 2 2 2 2 2 2 2 2 2 2 2 2 2 2 0 2 2 2 2 ( ) ( ) 1 ) sin ( .) ( ) (sin ( ) sin ( ) ( ) 1 ) sin ( ) (sin ( ) sin sin ( ) ( ) 1 ) ( ) (sin ( ) sin sin ( ) ( )sin 1 ) ( ) (sin ( )sin 1 ) ( .) 4 ( 8 ) ( ) ( ( ) 1 m let m eq multiplied r R eq r ze E h m r R r r R r r let e ] ( ) ( )sin 1 ) ( ) (sin ( )sin 1 ) [ 4 ( 8 ) ( ) ( ( ) 1 2 2 2 2 02 22 2 r r ze E h m r R r r R r r e Angular part Radial part
按次数下载不扣除下载券;
注册用户24小时内重复下载只扣除一次;
顺序:VIP每日次数-->可用次数-->下载券;
- 厦门大学:《结构化学 Structural Chemistry》课程教学资源(课件讲稿)Chapter 1 The Basic Knowledge of Quantum Mechanics(讲授:曹泽星、蒋亚琪).pdf
- 厦门大学:《量子化学 Quantum Chemistry》课程电子教案(教学课件)Chapter 9 多原子的半经验方法 Semi-experimental method for polyatomic molecular.ppt
- 厦门大学:《量子化学 Quantum Chemistry》课程电子教案(教学课件)Chapter 8 共轭分子的结构与性能 Ab initio technique for polyatomic molecular.pdf
- 厦门大学:《量子化学 Quantum Chemistry》课程电子教案(教学课件)Chapter 7 简单分子轨道理论 Elementary molecular orbital(MO)theory.pdf
- 厦门大学:《量子化学 Quantum Chemistry》课程电子教案(教学课件)Chapter 6 分子的对称性与对称群 Molecular symmetry and symmetric group.pdf
- 厦门大学:《量子化学 Quantum Chemistry》课程电子教案(教学课件)Chapter 5 原子结构 Atomic structure.pdf
- 厦门大学:《量子化学 Quantum Chemistry》课程电子教案(教学课件)Chapter 4 角动量与自旋 Angle momentum and spin.ppt
- 厦门大学:《量子化学 Quantum Chemistry》课程电子教案(教学课件)Chapter 3 矩阵与算符 Matrix and operator.ppt
- 厦门大学:《量子化学 Quantum Chemistry》课程电子教案(教学课件)Chapter 2 简单量子力学体系 Rudimental quantum mechanics system.ppt
- 厦门大学:《量子化学 Quantum Chemistry》课程电子教案(教学课件)Chapter 1 Schrödinger 方程 Schrodinger equation.ppt
- 中国科学技术大学:《高分子化学》课程教学资源(课件讲稿)第六、七、八、九章 离子聚合、开环聚合、链式共聚合反应、配位聚合、聚合物的化学反应(主讲:刘世勇).pdf
- 和频光谱在生物膜等生物界面中的应用 In situ molecular level studies on membrane related peptides and proteins in real time using sum frequency generation vibrational spectroscopy.pdf
- 上饶师范学院:《无机化学》课程电子教案(课件讲稿)第二十二章 镧系元素和锕系元素.pdf
- 上饶师范学院:《无机化学》课程电子教案(课件讲稿)第二十一章 过渡元素(二).pdf
- 上饶师范学院:《无机化学》课程电子教案(课件讲稿)第二十章 过渡元素(一).pdf
- 上饶师范学院:《无机化学》课程电子教案(课件讲稿)第十八章 铜、锌副族.pdf
- 上饶师范学院:《无机化学》课程电子教案(课件讲稿)第十七章 碱金属与碱土金属.pdf
- 上饶师范学院:《无机化学》课程电子教案(课件讲稿)第十四章 氮族元素.pdf
- 上饶师范学院:《无机化学》课程电子教案(课件讲稿)第十五章 碳族元素(碳、硅、硼).pdf
- 上饶师范学院:《无机化学》课程电子教案(课件讲稿)第十二章 卤素.pdf
- 厦门大学:《结构化学 Structural Chemistry》课程教学资源(课件讲稿)Chapter 3 Molecular symmetry and symmetry point group.pdf
- 厦门大学:《结构化学 Structural Chemistry》课程教学资源(课件讲稿)Chapter 4 The structure of diatomic molecules.pdf
- 厦门大学:《结构化学 Structural Chemistry》课程教学资源(课件讲稿)Chapter 5 The structure of polyatomic molecules.pdf
- 厦门大学:《结构化学 Structural Chemistry》课程教学资源(课件讲稿)Chapter 6 Polyatomic molecules(II).pdf
- 厦门大学:《群论及其在化学中的应用》课程教学资源(课件讲稿)Group Theory and Its Application to Quantum Chemistry - Introduction(主讲:曹泽星).pdf
- 厦门大学:《群论及其在化学中的应用》课程教学资源(课件讲稿)Part I 群论基础 Chapter 1 基本概念 Chapter 2 抽象群的结构.pdf
- 厦门大学:《群论及其在化学中的应用》课程教学资源(课件讲稿)Chapter 3 群的类分解 Chapter 4 商群与同态(Factor group & Homomorphism)Chapter 5 群的直积(Direct Product)Chapter 6 置换群(Permutation group/Symmetric group).pdf
- 厦门大学:《群论及其在化学中的应用》课程教学资源(课件讲稿)Chapter 7 Cayley定理 Chapter 8 线性向量空间(Linear vector spaces)Chapter 9 线性算符(Linear Operator)Chapter 10 群的表示.pdf
- 厦门大学:《群论及其在化学中的应用》课程教学资源(课件讲稿)Chapter 11 酉空间(Unitary Space)Chapter 12 表示的约化及其判据 Chapter 13 正交定理 The Orthogonality Relations Chapter 14 直积群的表示 Chapter 15 表示的分解 Chapter 16 投影算符(Projection Operator).pdf
- 《普通化学》课程电子教案(PPT教学课件)第9章 仪器分析基础.pptx
- 山西师范大学:《生物化学》课程教学大纲.pdf
- 山西师范大学:《生物化学实验》课程教学大纲.pdf
- 中国石油大学(华东):《结构化学》课程电子教案(教学课件)绪论(主讲:任浩).pdf
- 中国石油大学(华东):《结构化学》课程电子教案(教学课件)第四章 分子结构.pdf
- 中国石油大学(华东):《结构化学》课程电子教案(教学课件)第一章 量子力学基础.pdf
- 中国石油大学(华东):《结构化学》课程电子教案(教学课件)第二章 运动的量子理论.pdf
- 中国石油大学(华东):《结构化学》课程电子教案(教学课件)第六章 固体.pdf
- 中国石油大学(华东):《结构化学》课程电子教案(教学课件)第三章 原子结构和原子光谱.pdf
- 中国石油大学(华东):《结构化学》课程电子教案(教学课件)第五章 对称性.pdf
- 安徽建筑大学:材料与化学工程学院应用化学专业培养方案(2019版).doc
