上海交通大学：《系统模型、分析与控制 Modeling、Analysis and Control》课程教学资源[05]Lecture41-Transfer function 传递函数

ME369-系统模型、分析与控制 4.1传递函数 School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 传递函数定义 传递函数(Transfer Function,TF): 在零初始条件下，线性定常系统输出量的 拉氏变换与引起该输出的输入量的拉氏变换之比。 输入 Gx】=X. X,(s) [x,(t】X,(s) 线性定常系统 X.() 零初始条件(Zero initial conditions,(ZC) 输出 →输入量施加于系统之前，系统处于稳定的工作状态 (响应函数) x(t)=x(t)=元(t)=x(t)=.=0,1≤0 X(s)=X(s)G(s) →t(=0,输出量及其各阶导数也均为0： School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 1

1 ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 4.1 传递函数 ME 369– 系统模型、分析与控制 ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 传递函数（Transfer Function， TF)： 在零初始条件下，线性定常系统输出量的 拉氏变换与引起该输出的输入量的拉氏变换之比。 输入 [ ( )] ( ) ( ) [ ( )] ( )  c c r r L x t X s G s L x t X s X (s) c ( ) X s r 线性定常系统  输入量施加于系统之前，系统处于稳定的工作状态 X (s) X (s)G(s) c  r 传递函数定义 输出 (响应函数) ( ) ( ) ( ) ( ) ... 0, 0 cccc x t x t x t x t t       t <= 0 ,输出量及其各阶导数也均为0； 零初始条件（Zero initial conditions，(ZIC)

传递函数定义（续） d"x (t) d.() dtn +a1 d-x() d- +…+am- dt +a x(t) =x@+h4 dtm 0+ dt 2+bmx,(0 ZIC du dr (aos"+as"+..+as +a)Xe(s) =(bos"+bs"+.+ms +bm),(s) G(s)= Xe(s)bos"+bs"++omis +bm X,(s)）as”+as-+.+an-1s+an School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 传递函数定义（续） G(s)= Xe(s)bos"+bs"++mis +bm X(s)dos"+as"+..+as +an G0)=6=K a 系统的放大系数(amplification coefficient)或增益(gain) School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 2

2 ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University n n n n m m m m r c a s a s a s a b s b s b s b X s X s G s               1 1 0 1 1 1 0 1 ... ... ( ) ( ) ( ) 1 0 1 1 1 0 1 1 ( ... ) ( ) ( ... ) ( ) n n n n c m m m m r a s a s a s a X s b s b s b s b X s              ( ) ( ) ... ( ) ( ) ( ) ( ) ... ( ) ( ) 1 1 1 0 1 1 1 1 0 1 b x t dt d x t b dt d x t b dt d x t b a x t dt dx t a dt d x t a dt d x t a m r r m m r m m r m n c c n n c n n c n                L[] ( )i i d dt i ZIC s 传递函数定义（续） ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University n n n n m m m m r c a s a s a s a b s b s b s b X s X s G s               1 1 0 1 1 1 0 1 ... ... ( ) ( ) ( ) (0) m n b G a   K 系统的放大系数（amplification coefficient）或增益（gain） 传递函数定义（续）

传递函数定义（续） x,(t)=6(t) 一X(s)=L[6(t)]=1 x.(t) X(s) G(s)= X（⑤=X.(s)=[g】 X,(s) 系统的脉冲响应函数(Impulse--Response Function) School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 例1]求传递函数 0 0 M+B d0_血， dr +K[玉)-=f) d在0，) K()x+)=0 ● School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 3

3 ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University ( ) ( ) ( ) [ ] ( ) ( ) c c r X s G s X s L s g t X    x (t) (t) r   x (t) c Xr (s)  L[ (t)] 1 X (s) c 系统的脉冲响应函数（Impulse-Response Function） 传递函数定义（续） ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University [例1]求传递函数   2 1 1 2 1 1 1 2 2 ( ) ( ) ( ) ( ) ( ) ( ) d x t dx t dx t M B K x t x t f t dt dt dt              2 2 1 2 2 1 1 2 2 2 2 ( ) ( ) ( ) ( ) ( ) ( ) 0 d x t dx t dx t M B K x t x t K x t dt dt dt            

传递函数定义（续） Gg)=bs”+bs1++bs+b2 dos"+a s+.+nis +an Z(i=1,2,…,m) b(s-3)s-22）(s-2m) 取决于 Gs)= 系统结构参数 a(s-2s-22)(s-2） 特征式/特征方程 P;(j=1,2,…,n) 系统的特征根 动态特性 School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fa12015 传递函数定义（续） 设v个零极点 v+d+2e n d个实极点 P,=-B b+2c=m (不含零极点) 1 -B=8+月=元，+D b个实零点 21=-0 S-2=s+0,=二(t,S+1) T, d对共轭极点 P,=-a,±jy 6+a-mXs+a+m)=2+25.1s+)） 1 c对共轭复零点 2=-a±jy, (s+a-InXs+a+j)=(ris+25s+1) School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 4

4 ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University ( )( )...( ) ( )( )...( ) ( ) 0 1 2 0 1 2 n m a s p s p s p b s z s z s z G s        n n m n m m m m a s a s a s a b s b s b s b G s              1 1 0 1 1 1 0 1 ... ... ( ) Zi (i=1, 2, …, m) Pj (j=1, 2, …, n) 系统的特征根 特征式/特征方程 动态特性 取决于 系统结构参数 传递函数定义（续） ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University v个零极点 d个实极点 （不含零极点） b个 实零点 d 对共轭极点 c 对共轭复零点 v+d+2e = n b+2c = m 设 i i z   pj j   1 i i i ( )1 i s z s s         1 ( )1 j j j j s p s T s T       i i i z a j     2 2 2 1 ( )( ) ( 2 )        1         i i i i ai ai ai ai s j s j s s i i i p a j     2 2 2 1 ( )             ( ) ( 2 )1 i i i i ni ni ni ni s j s j T s T s T 传递函数定义（续）

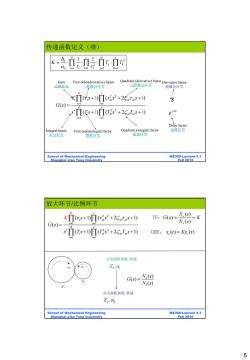
传递函数定义（续） K-1 ao i=1 =1 i=1 Gain First order(derivative)factor Quadratic(derivative)factor Derivative factor 比例环节 一阶微分环节 二阶微分环节 纯微分环节 K(+l)r后s2+2sras+） S G(s)= 1= s'Π(Ts+lΠT2+25Ims+) e i=1 =1 Delay factor Integral factor First order(integral)factor Quadratic (integral)factor 延滞环节 积分环节 惯性环节 振荡环节 School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 放大环节/比例环节 KII(ts+D)II(+2+) TF:G(s)= X(s)=K G(s)= X,(s) s'(Ts+(T2s2+25Ts+1) ODE:x(t)=Kx,(t) i=1 主动齿轮齿数，转速 Zm N2(s) Z G(s)= N(s) 从动齿轮齿数，转速 Z2,n3 School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 5

5 ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University Gain 比例环节 First order(derivative) factor 一阶微分环节 Quadratic (derivative) factor 二阶微分环节 Integral factor 积分环节 First order(integral) factor 惯性环节 Quadratic (integral) factor 振荡环节 Delay factor 延滞环节 Derivative factor 纯微分环节 2 2 1 1 2 2 1 1 ( ) ( 2 ) ( ) ( ) ( 2 ) 1 1 1 1 b c i ai ai ai i i d e v i ni ni ni i i K s s s G s s T s T s T s                     s s e               e k k d j j c l l b i i T T a b K 1 2 1 1 2 0 1 0 1 1   传递函数定义（续） ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 放大环节/比例环节 x (t) Kx (t) c  r ( ) ( ) ( ) c r X s G X s K s   ODE： 2 2 TF： 1 1 2 2 1 1 ( 1) ( 2 1) ( ) ( 1) ( 2 1) b c i ai ai ai i i d e v i ni ni ni i i s s s G s s T s T s T s K                     2 1 ( ) ( ) ( ) N s G s N s  1 1 Z n, 主动齿轮齿数,转速 2 2 Z n, 从动齿轮齿数,转速

惯性环节 KII(s+DTI(+25+D) G(s)= '门s+(T2+25Is+1) X(s)K 环节的放大系数(Gain) 传递函数 G(s)= X.(s)Ts+1 环节的时间常数(time constant) 运动微分方程 T@+x0=,0 dt School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 例2]惯性环节 x(t) 0 k(r-y)=B t 初始位置 B B dy +ky=kx dt G(s)= Y(s) X(s) School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 6

6 ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University ( ) ( ) ( ) x t Kx t dt dx t T c r c   ( ) ( ) ( ) 1 c r X s G s X s s K T    运动微分方程 传递函数 2 2 1 1 2 2 1 1 ( 1) ( 2 1) ( ) ( 1) ( 2 1) b c i ai ai ai i i d e v ni ni ni i i i K s s s G s s T s s T s T                     环节的放大系数（Gain） 环节的时间常数（time constant） 惯性环节 ME369-Lecture 4.1 Fall 2015 Initial position 初始位置 School of Mechanical Engineering Shanghai Jiao Tong University d ( ) d y k x y B t   d d y B ky kx t   ( ) ( ) ( ) Y s G s X s  [例2]惯性环节

[例3]惯性环节 以=Ri+d G(s)= U.(s) U,(s) School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 积分环节 Kr+门r2s2+25arrs+l0 G(s)= s+7*+25.s+) 传递函数： G(s)= X.(s)K X(s)s 运动方程式： 0=Kx,0一0=Kxh dt School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 7

7 ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 1 d r u Ri i t C    1 d c u i t C   ( ) ( ) ( ) c r U s G s U s  [例3]惯性环节 ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University ( ) ( ) c r dx t Kx t dt  ( ) ( ) ( ) c r X s G s X s K s   运动方程式： 传递函数：   t c r x t K x t dt 0 ( ) ( ) 2 2 1 1 2 2 1 1 ( 1) ( 2 1) ( ) ( 1) ( 2 1) b c i ai ai ai i i d e i ni ni ni i v i K s s s G s s T s T s T s                     积分环节

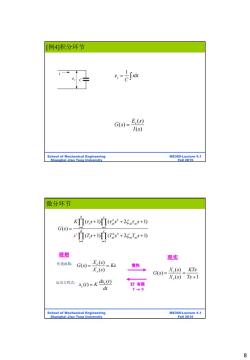
[例4]积分环节 e.fidr G(s)= E.(s) I(s) School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 微分环节 KTI5+DT+25+) G(s)= 1+s+257.5+0 理想 现实 传递函数： G(s)= X(5)=Ks 惯性 X,(s) X(s)KTs G(s)= X(s)Ts+1 运动方程式： ()=K在) XT有限 dt T→0 School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 8

8 ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 1 d c e i t C   ( ) ( ) ( ) E s c G s I s  [例4]积分环节 ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 微分环节 理想 dt dx t x t K r c ( ) ( )  Ks X s X s G s r c   ( ) ( ) ( ) 运动方程式： 传递函数： 现实 ( ) 1 ( ) ( )    Ts KTs X s X s G s r c 2 2 1 1 2 2 1 1 ( 1) ( 2 1) ( ) ( 1) ( 2 1) b c i ai ai ai i i d e i ni ni ni i v i K s s s G s s T s T s T s                     惯性 KT 有限 T  0

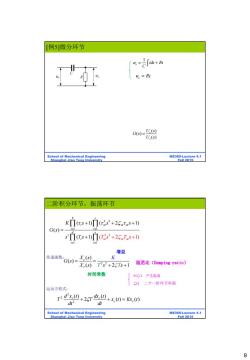
[例5]微分环节 +危 u。=Ri G(s)= U(s) U,(s) School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 阶积分环节，振荡环节 K(rs+li(rs2+25ras+） G(s)= s'(s+1i(Ts2+25s+1） 增益 传递函数： K G(s)= X(s) X,(s) T2s2+25Ts+1 阻尼比(Damping ratio) 时间常数 0<化<1产生振荡 21二个一阶环节串联 运动方程式： T2dx0+2T.@ di2 +x.(t)=K(t) dt School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 9

9 ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University [例5]微分环节 1 d r u i t Ri C    c u Ri  ( ) ( ) ( ) c r U s G s U s  ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University ( ) ( ) ( ) 2 ( ) 2 2 2 x t Kx t dt dx t T dt d x t T c r c c     2 2 ( ) ( ) ( ) 2 1 c r X s K G s X s s T Ts     0<<1 产生振荡 1 二个一阶环节串联 2 2 2 1 1 1 1 2 ( 1) ( 2 ( 2 1) 1) ( ) ( 1) b c i ai ai ai i i d e v i i ni n i ni i K s T s T s s G s s T s s                     增益 时间常数 阻尼比（Damping ratio） 二阶积分环节，振荡环节 运动方程式： 传递函数：

二阶积分环节，振荡环节（续） R u,(t) 白Bx MKx()=) LCdu()+RCdu.() +u.(t)=u(t) dr2 dr d _U(s) 1 G(s)= (s)_ 1/K G(s)= (s) + B U,(s)LCs2+RCs+1 s+1 K 不同的情能元件 能壹转换 振满 School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fa12015 阶微分环节 KHIGS+DI+26+D G(s)= ss+72+25.7s+l i= 传递函数： 增益 G(s)= X(回=K(r232+25rs+1) X,(s) 时间常数 ≥1二个一阶环节串联 运动方程式： 30)=KIrd's (25) dr2 di x,(t】 School of Mechanical Engineering ME369-Lecture 4.1 Shanghai Jiao Tong University Fall 2015 10

10 ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University 2 2 ( ) ( ) ( ) ( ) d x t dx t M B Kx t f t dt dt    2 2 d ( ) d ( ) ( ) ( ) d d c c c r u t u t LC RC u t u t t t    2 ( ) 1/ ( ) ( ) 1 X s K G s F s M B s s K K     2 ( ) 1 ( ) ( ) 1 c r U s G s U s LCs RCs     不同的储能元件 能量转换 振荡 二阶积分环节，振荡环节（续） ME369-Lecture 4.1 Fall 2015 School of Mechanical Engineering Shanghai Jiao Tong University ( )] ( ) 2 ( ) ( ) [ 2 2 2 x t dt dx t dt d x t x t K r r r c      2 2 ( ) ( ) ( 2 1) ( ) c r X s G s s s X s     K   2 2 1 2 1 1 2 1 ( 1) ( 2 ( ) ( 1) ( 2 1) 1) b c i i i d e v i ai ai ni ni ni i i K s ai G s s T s s T s T s     s                 运动方程式： 传递函数： 1 二个一阶环节串联 增益 时间常数 二阶微分环节