《电磁学》课程教学资源（拓展资料）Exam and Solutions Exam 2 Practice Problems Part 1 Solutions

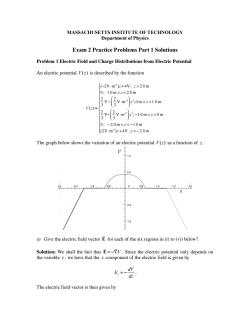
MASSACHUSETTS INSTITUTE OF TECHNOLOGY Department of Physics Exam 2 Practice Problems Part 1 Solutions Problem 1 Electric Field and Charge Distributions from Electric Potential An electric potential V(z)is described by the function (-2Vm)z+4V;z>2.0m 0；1.0m<z<2.0m 八3 (2 Vm33,0m<z<1.0m 3 V(z)= 2 V+ 2vm3-10m<:<0m 3 0；-2.0m<z<-1.0m (2Vm)z+4V;z<-2.0m The graph below shows the variation of an electric potential V()as a function of z. V 1.6 08 -24 -16 08 0 08 1.6 24 3 -0.8 -1.6 a)Give the electric field vector E for each of the six regions in (i)to (vi)below? Solution:We shall the fact that E=-VV.Since the electric potential only depends on the variable z,we have that the z-component of the electric field is given by Es、d业 dz The electric field vector is then given by

MASSACHUSETTS INSTITUTE OF TECHNOLOGY Department of Physics Exam 2 Practice Problems Part 1 Solutions Problem 1 Electric Field and Charge Distributions from Electric Potential An electric potential V (z) is described by the function V (z) = (!2V"m-1 )z + 4V ; z > 2.0 m 0 ; 1.0 m < z < 2.0 m 2 3 V ! 2 3 V"m-3 # $ % & ' ( z3 ; 0 m < z <1.0 m 2 3 V+ 2 3 V"m-3 # $ % & ' ( z3 ; !1.0 m < z < 0 m 0 ; ! 2.0 m < z < !1.0 m (2V"m-1 )z + 4V ; z < ! 2.0 m ) * + + + + + , + + + + + The graph below shows the variation of an electric potential V (z) as a function of z . a) Give the electric field vector E ! for each of the six regions in (i) to (vi) below? Solution: We shall the fact that E = !"V ! ! . Since the electric potential only depends on the variable z , we have that the z -component of the electric field is given by z dV E dz = ! . The electric field vector is then given by

E=E.i=-dVk dz (iz>2.0m: E=-dk=-4(-2V-m)z+4v)k=2V.m8 dz d (ii)1.0m0 is a very small number is given by E-=(2V.mX(lm)k=2Vk m Note that the z-component of the electric field,2 V.m,has the correct units. (iv)-1.0m<z<0m: E=vm月 =-(2Vm）k The value of the electric field at a point just inside z*=-1.0 m+g m is given by E-=-(2V.mXlm)k=-2Yk. m (v)-2.0m<z<-1.0m: E=-k=0 d (vi)z<-2.0m: EV.m)+4V)--2V-m'k dz

ˆ ˆ z dV E dz E = i = ! k ! (i) z > 2.0 m : ( ) -1 -1 ˆ ˆ ˆ ( 2V m ) 4V 2V m dV d z dz dz E = ! k = ! ! " + k = " k ! (ii) 1.0 m 0 is a very small number is given by ! E! = (2 V"m-3 )(1m) 2 kˆ = 2 V m kˆ Note that the z-component of the electric field, 2 V!m"1 , has the correct units. (iv) −1.0 m 0 m < <z : ! E = ! dV dz kˆ = ! d dz 2 3 V+ 2 3 V"m-3 # $ % & ' ( z 3 # $ % & ' ( kˆ = ! 2 V"m-3 ( )z 2 kˆ The value of the electric field at a point just inside z+ = !1.0 m + " m is given by ! E! = !(2 V"m-3 )(1m) 2 kˆ = !2 V m kˆ . (v) −2.0 m 1.0 m < <z − : ˆ dV dz E = ! k = 0 ! ! (vi) z < ! 2.0 m : ( ) -1 -1 ˆ ˆ ˆ (2V m ) 4V 2V m dV d z dz dz E = ! k = ! " + k = ! " k !

b)Make a plot of the z-component of the electric field,E.,as a function of z.Make sure you label the axes to indicate the numeric magnitude of the field. E:2 2.0 [V/m].e 08 04 08 12 18 24 z m -2.0 c)Qualitatively describe the distribution of charges that gives rise to this potential landscape and hence the electric fields you calculated.That is,where are the charges, what sign are they,what shape are they (plane,slab...)? In the region -1.0 m2.0 m (hence the positive component of the electric field). On the plane =1.0 m,there is a negative uniform charge density -o. In the region -2.0 m<z<-1.0 m there is a conductor where the field is zero. On the plane z=-2.0 m,there is a positive uniform charge density o that produces a constant field pointing to the left in the region z<-2.0 m (hence the positive component of the electric field). On the plane z=-1.0 m,there is a negative uniform charge density -o

b) Make a plot of the z-component of the electric field, Ez , as a function of z. Make sure you label the axes to indicate the numeric magnitude of the field. c) Qualitatively describe the distribution of charges that gives rise to this potential landscape and hence the electric fields you calculated. That is, where are the charges, what sign are they, what shape are they (plane, slab…)? In the region !1.0 m 2.0 m (hence the positive component of the electric field). On the plane z =1.0 m , there is a negative uniform charge density "! . In the region !2.0 m < z < !1.0 m there is a conductor where the field is zero. On the plane z = ! 2.0 m , there is a positive uniform charge density ! that produces a constant field pointing to the left in the region z < ! 2.0 m (hence the positive component of the electric field). On the plane z = !1.0 m, there is a negative uniform charge density "!

Problem 2:Electric Field from Electric Potential The electric potential V(x)for a planar charge distribution is given by: 0 forxd where -V is the potential at the origin and d is a distance. V(x) 0 25 This function is plotted to the right,with d=2cm and =2V,the x-axis with units in cm,the y-axis in units of Volts. (a)What is the electric field E(x)for this problem? Region I:x<-d E=-7v=- vi=0 Region II:-d≤x<0 -

Problem 2: Electric Field from Electric Potential The electric potential V (x) for a planar charge distribution is given by: V (x) = 0 for x d ) * + + + + , + + + + where !V0 is the potential at the origin and d is a distance. This function is plotted to the right, with d = 2 cm and V0 = 2 V , the x-axis with units in cm, the y-axis in units of Volts. (a) What is the electric field ! E(x) for this problem? Region I: x < -d ! E = !"V = ! #V #x ˆ i = 0 Region II: !d " x < 0 ! E = ! " "x !V0 1+ x d # $ % & ' ( 2 # $ % % & ' ( ( ˆ i = 2 V0 d 1+ x d # $ % & ' ( ˆ i

Region III:0≤xd =-VV= avi-0 Ox (b)Plot the electric field that you just calculated on the graph below.Be sure to properly label the y-axis(top and bottom)to indicate the limits of the magnitude of the E field! E,(x) This makes sense.Where the potential is flat,the E field is 0

Region III: 0 ! x d ! E = !"V = ! #V #x ˆ i = 0 (b) Plot the electric field that you just calculated on the graph below. Be sure to properly label the y-axis (top and bottom) to indicate the limits of the magnitude of the E field! This makes sense. Where the potential is flat, the E field is 0

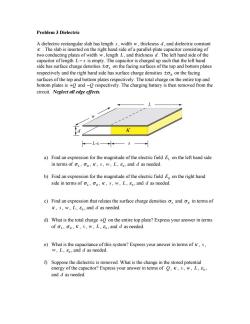
Problem 3 Dielectric A dielectric rectangular slab has length s,width w,thickness d,and dielectric constant K.The slab is inserted on the right hand side of a parallel-plate capacitor consisting of two conducting plates of width w,length L,and thickness d.The left hand side of the capacitor of length L-s is empty.The capacitor is charged up such that the left hand side has surface charge densities +o,on the facing surfaces of the top and bottom plates respectively and the right hand side has surface charge densities tog on the facing surfaces of the top and bottom plates respectively.The total charge on the entire top and bottom plates is +0 and-O respectively.The charging battery is then removed from the circuit.Neglect all edge effects. Ls一s→ a)Find an expression for the magnitude of the electric field E on the left hand side in terms of o,og,K,s,w,L,and d as needed b)Find an expression for the magnitude of the electric field Eg on the right hand side in terms of o.og,K,s,w,L,8,and d as needed. c)Find an expression that relates the surface charge densities o,and og in terms of K,s,w,L,8,and d as needed. d)What is the total charge +0 on the entire top plate?Express your answer in terms of oL,oR,K,s,w,L,Eo,and d as needed. e)What is the capacitance of this system?Express your answer in terms of K,s, w,L,and d as needed. f)Suppose the dielectric is removed.What is the change in the stored potential energy of the capacitor?Express your answer in terms of K,s,w,L,E, and d as needed

Problem 3 Dielectric A dielectric rectangular slab has length s, width w , thickness d , and dielectric constant ! . The slab is inserted on the right hand side of a parallel-plate capacitor consisting of two conducting plates of width w , length L , and thickness d . The left hand side of the capacitor of length L ! s is empty. The capacitor is charged up such that the left hand side has surface charge densities ±! L on the facing surfaces of the top and bottom plates respectively and the right hand side has surface charge densities ±! R on the facing surfaces of the top and bottom plates respectively. The total charge on the entire top and bottom plates is +Q and !Q respectively. The charging battery is then removed from the circuit. Neglect all edge effects. a) Find an expression for the magnitude of the electric field EL on the left hand side in terms of ! L , ! R , ! , s, w , L , ! 0 , and d as needed. b) Find an expression for the magnitude of the electric field ER on the right hand side in terms of ! L , ! R , ! , s, w , L , ! 0 , and d as needed. c) Find an expression that relates the surface charge densities ! L and ! R in terms of ! , s, w , L , ! 0 , and d as needed. d) What is the total charge +Q on the entire top plate? Express your answer in terms of ! L , ! R , ! , s, w , L , ! 0 , and d as needed. e) What is the capacitance of this system? Express your answer in terms of ! , s, w , L , ! 0 , and d as needed. f) Suppose the dielectric is removed. What is the change in the stored potential energy of the capacitor? Express your answer in terms of Q , ! , s, w , L , ! 0 , and d as needed

Solutions: a)Find an expression for the magnitude of the electric field E,on the left hand side in terms of o,og,K,s,w,L,and d as needed. Using Gauss's LawE b)Find an expression for the magnitude of the electric field Eg on the right hand side in terms of o,og,K,s,w,L,8,and d as needed. Using Gauss's Law for dielectrics E E c)Find an expression that relates the surface charge densities o,and og in terms of K,s,w,L,and d as needed. The potential differencen the le side isEOn the right hand side it is Ed Since these must be equal we must have E。 d)What is the total charge +0 on the entire top plate?Express your answer in terms of o,og,K,s,w,L,E,and d as needed. =0(L-s)w+oRSw e)What is the capacitance of this system?Express your answer in terms of K,s, w,L,o,and d as needed. The potential difference is Edd -so the capacitance is C=_ -2u-[u-4]-c od f)Suppose the dielectric is removed.What is the change in the stored potential energy of the capacitor?Express your answer in terms of K,s,w,L,8, and d as needed. Since the battery has been removed,the charge on the capacitor does not change when we do this.So the change in the energy stored is

Solutions: a) Find an expression for the magnitude of the electric field EL on the left hand side in terms of ! L , ! R , ! , s, w , L , ! 0 , and d as needed. Using Gauss’s Law EL = ! L " o b) Find an expression for the magnitude of the electric field ER on the right hand side in terms of ! L , ! R , ! , s, w , L , ! 0 , and d as needed. Using Gauss’s Law for dielectrics R R o E σ κε = c) Find an expression that relates the surface charge densities ! L and ! R in terms of ! , s, w , L , ! 0 , and d as needed. The potential difference on the left side is L L o d E d σ ε = . On the right hand side it is R R o d E d σ κε = . Since these must be equal we must have R L σ σ κ = d) What is the total charge +Q on the entire top plate? Express your answer in terms of ! L , ! R , ! , s, w , L , ! 0 , and d as needed. Q L s w sw =σ σ L R ( − ) + e) What is the capacitance of this system? Express your answer in terms of ! , s, w , L , ! 0 , and d as needed. The potential difference is L L o d E d σ ε = , so the capacitance is ( ) ( ) ( ) L R o o R L L o Q L s w sw w w C Ls s Ls s C V dd d σ σ ε ε σ κ σ σ ε − + ⎡ ⎤ == = ⎢ ⎥ − + = ⎡ ⎤ − + = ⎣ ⎦ ∇ ⎣ ⎦ f) Suppose the dielectric is removed. What is the change in the stored potential energy of the capacitor? Express your answer in terms of Q , ! , s, w , L , ! 0 , and d as needed. Since the battery has been removed, the charge on the capacitor does not change when we do this. So the change in the energy stored is

enag是-是-- d e定g亿-+sg4H 1 28w L (L-s)+ks change in energy= oa( od (K-1)s 2ew[[(L-s)+]

( ) ( ) 2 22 2 2 1 1 11 change in energy 22 2 1 1 2 2 o o o o o Q QQ C C CC Q d d Qd ε εκ Lw w L L s s ε κ wLs s ⎛ ⎞ = − = ⎜ ⎟ − ⎝ ⎠ ⎛ ⎞ ⎡ ⎤ = ⎜ ⎟ − = ⎢ ⎥ − ⎡ ⎤ − + − + ⎝ ⎠ ⎣ ⎦ ⎣ ⎦ ( ) ( ) ( ) ( ) 2 2 1 change in energy 2 2 o o Qd Qd Ls sL s w w LLs s LLs s κ κ ε ε κ κ ⎡ ⎤⎡ ⎤ − + − − = = ⎢ ⎥⎢ ⎥ ⎢ ⎥⎢ ⎥ ⎡ ⎤⎡ ⎤ ⎡⎤ ⎡⎤ − + − + ⎣ ⎦⎣ ⎦ ⎣ ⎦⎣ ⎦ ⎣⎦ ⎣⎦

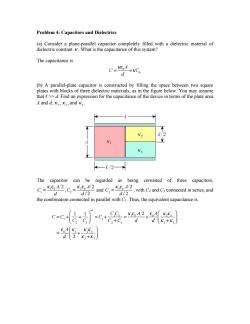
Problem 4:Capacitors and Dielectrics (a)Consider a plane-parallel capacitor completely filled with a dielectric material of dielectric constant k.What is the capacitance of this system? The capacitance is C=K d -=KCo (b)A parallel-plate capacitor is constructed by filling the space between two square plates with blocks of three dielectric materials,as in the figure below.You may assume that t>>d.Find an expression for the capacitance of the device in terms of the plate area A and d,K,K,,and K3. K2 d/2 K 业 K3 —0/2 The capacitor can be regarded as being consisted of three capacitors, C-K6 4/2.C,=K 4/2 d d/2 and C=Ke 4/2 with C and C3 conected in series,and the combination connected in parallel with C1.Thus,the equivalent capacitance is c-c-c+62 +

Problem 4: Capacitors and Dielectrics (a) Consider a plane-parallel capacitor completely filled with a dielectric material of dielectric constant κ . What is the capacitance of this system? The capacitance is C = !" 0A d =!C0 (b) A parallel-plate capacitor is constructed by filling the space between two square plates with blocks of three dielectric materials, as in the figure below. You may assume that ℓ >> d. Find an expression for the capacitance of the device in terms of the plate area A and d, ! 1 , ! 2 , and ! 3 . The capacitor can be regarded as being consisted of three capacitors, C1 = ! 1 " 0 A 2 d ,C2 = ! 2 " 0 A 2 d / 2 and C3 = ! 3 " 0 A 2 d / 2 , with C2 and C3 connected in series, and the combination connected in parallel with C1. Thus, the equivalent capacitance is C = C1 + 1 C2 + 1 C3 ! " # $ % & '1 = C1 + C2 C3 C2 + C3 = ( 1 ) 0 A 2 d + ) 0A d ( 2 ( 3 ( 2 +( 3 ! " # $ % & = ) 0A d ( 1 2 + ( 2 ( 3 ( 2 +( 3 ! " # $ % &

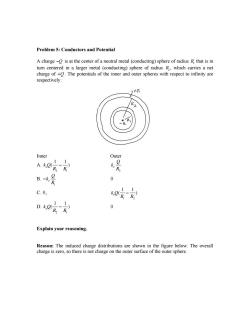
Problem 5:Conductors and Potential A charge-O is at the center of a neutral metal(conducting)sphere of radius R that is in turn centered in a larger metal (conducting)sphere of radius R,which carries a net charge of +O.The potentials of the inner and outer spheres with respect to infinity are respectively: +6 01 - Inner Outer A.kO( 1 B.-k B Q 0 C.0, R D.k.O( 1 0 ,R Explain your reasoning. Reason:The induced charge distributions are shown in the figure below.The overall charge is zero,so there is not charge on the outer surface of the outer sphere

Problem 5: Conductors and Potential A charge !Q is at the center of a neutral metal (conducting) sphere of radius R1 that is in turn centered in a larger metal (conducting) sphere of radius R2 , which carries a net charge of +Q. The potentials of the inner and outer spheres with respect to infinity are respectively: Inner Outer A. ke Q( 1 R2 ! 1 R1 ) ke Q R2 B. !ke Q R1 0 C. 0, ke Q( 1 R1 ! 1 R2 ) D. ke Q( 1 R2 ! 1 R1 ) 0 Explain your reasoning. Reason: The induced charge distributions are shown in the figure below. The overall charge is zero, so there is not charge on the outer surface of the outer sphere