香港中文大学：《Probability and Statistics for Engineers》课程教学资源（辅导材料）Tutorial 4：Discrete Random Variables 2

Tutorial 4:Discrete Random Variables 2 Baoxiang Wang bxwang@cse Spring 2017

Tutorial 4: Discrete Random Variables 2 Baoxiang Wang bxwang@cse Spring 2017 1

Geometric Random variables The PMF of a geometric random variable is px(k)=(1-p)k-1p ·EXX>1]=1+E[X]

Geometric Random Variables • The PMF of a geometric random variable is 𝑝𝑋 𝑘 = (1 − 𝑝) 𝑘−1𝑝 • 𝐸 𝑋 𝑋 > 1 = 1 + 𝐸[𝑋] 2

Geometric Random variables 。Fork>1, P(X=kx >1)= P(X=k 2=_(1-p)k-1p (1-p)k-1p P(X>1) 280-2(1-p)k-1p -p(1-p)1-4-p 1 =(1-p)k-2p=px(k-1) ·EXx>1]=∑K=2kg(X=kX>1) -∑px-1)=∑k+1pr侧=∑px+∑x个 =子E[X] k=1 k=1 3

Geometric Random Variables • For 𝑘 > 1, 𝑃 𝑋 = 𝑘 𝑋 > 1 = 𝑃(𝑋=𝑘) 𝑃(𝑋>1) = (1−𝑝) 𝑘−1𝑝 σ𝑘=2 ∞ (1−𝑝) 𝑘−1𝑝 = (1−𝑝) 𝑘−1𝑝 𝑝(1−𝑝)∙ 1 1−(1−𝑝) = (1 − 𝑝) 𝑘−2𝑝 = 𝑝𝑋(𝑘 − 1) • 𝐸 𝑋 𝑋 > 1 = σ𝑘=2 ∞ 𝑘𝑃 𝑋 = 𝑘 𝑋 > 1 = ෍ 𝑘=2 ∞ 𝑘𝑝𝑋(𝑘 − 1) = ෍ 𝑘=1 ∞ (𝑘 + 1)𝑝𝑋(𝑘) = ෍ 𝑘=1 ∞ 𝑘𝑝𝑋(𝑘) + ෍ 𝑘=1 ∞ 𝑝𝑋(𝑘) = 1 + 𝐸[𝑋] 3

Geometric Random variables 。In general,, P(X=k) PX=k X>a= P(X>a) (1-p)k-1p (1-p)k-1p 2R=a+1(1-p)k-1p 1 1 (1-p)a.1-(1-p) =(1-p)k-a-1p =px(k-a)

Geometric Random Variables • In general, 𝑃 𝑋 = 𝑘 𝑋 > 𝑎 = 𝑃(𝑋 = 𝑘) 𝑃(𝑋 > 𝑎) = (1 − 𝑝) 𝑘−1𝑝 σ𝑘=𝑎+1 ∞ (1 − 𝑝) 𝑘−1𝑝 = (1 − 𝑝) 𝑘−1𝑝 𝑝(1 − 𝑝) 𝑎∙ 1 1 − (1 − 𝑝) = (1 − 𝑝) 𝑘−𝑎−1𝑝 = 𝑝𝑋(𝑘 − 𝑎) 4

Geometric Random variables .E [fX)X>a]=E[f(X a)] ·E[f(X)IX>a] =∑fk)P(x=kIx>a)=∑fk)pxk-a) k=a+1 k=a+1 00 -〉∑fk+a)px()=Ef(X+a k三1 5

Geometric Random Variables • 𝐸 𝑓 𝑋 𝑋 > 𝑎 = 𝐸[𝑓(𝑋 + 𝑎)] • 𝐸 𝑓 𝑋 𝑋 > 𝑎 = ෍ 𝑘=𝑎+1 ∞ 𝑓(𝑘)𝑃 𝑋 = 𝑘 𝑋 > 𝑎 = ෍ 𝑘=𝑎+1 ∞ 𝑓(𝑘)𝑝𝑋(𝑘 − 𝑎) = ෍ 𝑘=1 ∞ 𝑓(𝑘 + 𝑎)𝑝𝑋(𝑘) = 𝐸[𝑓(𝑋 + 𝑎)] 5

Example 1:Functions of random variables Let X be a random variable that takes value from 0 to 9 with equal probability 1/10. (a)Find the PMF of the random variable Y =X mod(3). X 0,3,6,9 1,47 2,5,8 Y 0 1 2 0=2/5 3/10 3/10 6

Example 1: Functions of random variables • Let 𝑋 be a random variable that takes value from 0 to 9 with equal probability 1/10. • (a) Find the PMF of the random variable 𝑌 = 𝑋 𝑚𝑜𝑑(3). 6 X 0,3,6,9 1,4,7 2,5,8 Y 0 1 2 P 4 10 = 2/5 3/10 3/10

Example 1:Functions of random variables Let X be a random variable that takes value from 0 to 9 with equal probability 1/10. .(b)Find the PMF of the random variable Y 5 mod(X+1). X 0 3 A 5 6 8 0 2 1 0 5 5 5 5 0 1 2 5 6=15 品=5 1/10 10=1/2

Example 1: Functions of random variables • Let 𝑋 be a random variable that takes value from 0 to 9 with equal probability 1/10. • (b) Find the PMF of the random variable 𝑌 = 5 𝑚𝑜𝑑(𝑋 + 1). 7 X 0 1 2 3 4 5 6 7 8 9 Y 0 1 2 1 0 5 5 5 5 5 Y 0 1 2 5 P 2 10 = 1/5 2 10 = 1/5 1/10 5 10 = 1/2

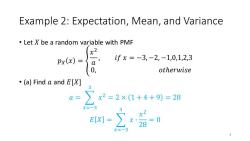
Example 2:Expectation,Mean,and Variance Let X be a random variable with PMF (x2 Px(x)= ifx=-3,-2,-1,0,1,2,3 (0, otherwise ·(a)Find a and E[X] a=∑x2=2×(1+4+9)=28 X二-3 X=-3 8

Example 2: Expectation, Mean, and Variance • Let 𝑋 be a random variable with PMF 𝑝𝑋 𝑥 = ൞ 𝑥 2 𝑎 , 𝑖𝑓 𝑥 = −3, −2, −1,0,1,2,3 0, 𝑜𝑡ℎ𝑒𝑟𝑤𝑖𝑠𝑒 • (a) Find 𝑎 and 𝐸[𝑋] 𝑎 = ෍ 𝑥=−3 3 𝑥 2 = 2 × 1 + 4 + 9 = 28 𝐸 𝑋 = ෍ 𝑥=−3 3 𝑥 ∙ 𝑥 2 28 = 0 8

Example 2:Expectation,Mean,and Variance Let X be a random variable with PMF x2 ifx=-3,-2,-1,0,1,2,3 otherwise .(b)What is the PMF of the random variable Z=(X-E[X])2? Z=X2 X 0 -11 2,2 3,3 Z 0 1 4 9 0 1 42 99 2×28=1/14 2×28-7 2×28=14 9

Example 2: Expectation, Mean, and Variance • Let 𝑋 be a random variable with PMF 𝑝𝑋 𝑥 = ൞ 𝑥 2 𝑎 , 𝑖𝑓 𝑥 = −3, −2, −1,0,1,2,3 0, 𝑜𝑡ℎ𝑒𝑟𝑤𝑖𝑠𝑒 • (b) What is the PMF of the random variable 𝑍 = (𝑋 − 𝐸[𝑋]) 2 ? 𝑍 = 𝑋 2 9 X 0 -1,1 -2,2 -3,3 Z 0 1 4 9 P 0 2 × 1 28 = 1/14 2 × 4 28 = 2 7 2 × 9 28 = 9 14

Example 2:Expectation,Mean,and Variance Let X be a random variable with PMF (x2 w- ifx=-3,-2,-1,0,1,2,3 otherwise .(c)Using the result from part(b),find the variance of X. 2 999 varX)=E☑]=1×i4+4×气+9 14= 14 Z 0 1 4 9 0 0 2×1/28 2×4/28 2×9/28 =1/14 =2/7 =9/14 10

Example 2: Expectation, Mean, and Variance • Let 𝑋 be a random variable with PMF 𝑝𝑋 𝑥 = ൞ 𝑥 2 𝑎 , 𝑖𝑓 𝑥 = −3, −2, −1,0,1,2,3 0, 𝑜𝑡ℎ𝑒𝑟𝑤𝑖𝑠𝑒 • (c) Using the result from part (b), find the variance of 𝑋. 𝑣𝑎𝑟 𝑋 = 𝐸 𝑍 = 1 × 1 14 + 4 × 2 7 + 9 × 9 14 = 99 14 10 Z 0 1 4 9 P 0 2 × 1/28 = 1/14 2 × 4/28 = 2/7 2 × 9/28 = 9/14