《桥牌与博弈论》课程教学资源：An Analysis of the Law of Total Tricks

An Analysis of the Law of Total Tricks Matt Ginsberg,Eugene,OR In a recent"Bridge World"article(1996年9月号，On the Law of Total Tricks,Matt Ginsberg),I discussed the possibility of creating a computer bridge player based on the development of a fast double-dummy solver.Such a tool would have significant impact on bidding theory as well.Do you want to know if the Law of Total Tricks is valid when the two sides have 21 trumps between them?Generate 1,000 deals fitting that description,analyze them using the double-dummy solver,and you'‖know. This note gives the results of such an analysis.The Law says that the two sides,when playing in their longest suits,can take between them as many tricks as they have trumps.Thus if N/S have a nine-card fit and E/W have a ten-card fit,the two sides can take a total of 19 tricks in the two suits.Is this really a law,or just a vague rule of thumb Here is the answer, where we have selected randomly among trump suits of equal length but always assume that the contract is played from declarer's best side

An Analysis of the Law of Total Tricks Matt Ginsberg, Eugene, OR In a recent “Bridge World” article(1996 年 9 月号, On the Law of Total Tricks, Matt Ginsberg), I discussed the possibility of creating a computer bridge player based on the development of a fast double-dummy solver. Such a tool would have significant impact on bidding theory as well. Do you want to know if the Law of Total Tricks is valid when the two sides have 21 trumps between them? Generate 1,000 deals fitting that description, analyze them using the double-dummy solver, and you'll know. This note gives the results of such an analysis. The Law says that the two sides, when playing in their longest suits, can take between them as many tricks as they have trumps. Thus if N/S have a nine-card fit and E/W have a ten-card fit, the two sides can take a total of 19 tricks in the two suits. Is this really a law, or just a vague rule of thumb ? Here is the answer, where we have selected randomly among trump suits of equal length but always assume that the contract is played from declarer's best side

Length samples average error 14 46944 -0.15 0.63 The columns in the table mean the 15 47281 -0.14 0.64 following: 16 120525 0.10 0.70 17 102184 0.02 0.75 18 69792 -0.01 0.83 1.The left-hand column gives 19 37561 -0.22 0.87 the combined length in the 20 15845 -0.50 0.99 21 5041 -0.89 1.20 two suits. 22 1286 -1.31 1.48 2.The second figure is the 23 237 -1.78 1.83 24 45 -2.22 2.27 number of deals in the Total 446741 -0.05 0.75 sample that met the given conditions. 3.The third figure is the average difference between the number of tricks that can be taken by each side when playing in their longest suit(from the best side)and the combined lengths.Thus the -0.15 on the first line means that,on average,the two sides can take a total of 13.85 tricks (14-0.15).With a combined length of 16 cards,the two sides can take an average of 16.10 tricks. 4.The fourth figure is the average of the error in the Law.So even though the Law is off by only 0.15 tricks if the best fits are of 7 cards each,on any individual deal we can expect the Law to be off by a little more than half a

The columns in the table mean the following: 1. The left-hand column gives the combined length in the two suits. 2. The second figure is the number of deals in the sample that met the given conditions. 3. The third figure is the average difference between the number of tricks that can be taken by each side when playing in their longest suit (from the best side) and the combined lengths. Thus the −0.15 on the first line means that, on average, the two sides can take a total of 13.85 tricks (14 − 0.15). With a combined length of 16 cards, the two sides can take an average of 16.10 tricks. 4. The fourth figure is the average of the error in the Law. So even though the Law is off by only 0.15 tricks if the best fits are of 7 cards each, on any individual deal we can expect the Law to be off by a little more than half a Length samples average error 14 46944 −0.15 0.63 15 47281 −0.14 0.64 16 120525 0.10 0.70 17 102184 0.02 0.75 18 69792 −0.01 0.83 19 37561 −0.22 0.87 20 15845 −0.50 0.99 21 5041 −0.89 1.20 22 1286 −1.31 1.48 23 237 −1.78 1.83 24 45 −2.22 2.27 Total 446741 −0.05 0.75

trick--it just overestimates approximately as often as it underestimates. To evaluate the specific actions suggested by the Law,we need a bit more detail.Here it is: Error Length samples -4-3-2-1 0 +1 +2+3 +4 14 46,9440.10.64.827.046.618.22.40.20.0 15 47,2810.10.65.026.645.719.22.60.20.0 16 120,5250.10.53.820.742.426.35.50.70.1 17 102,1840.10.65.223.539.424.55.80.70.1 18 69,7920.10.87.125.036.022.86.91.20.1 19 37,5610.11.310.728.933.818.85.41.00.1 20 15,8450.23.116.431.529.913.74.40.70.0 21 5,0410.77.424.031.923.310.12.30.30.0 22 1,2862.713.728.630.217.75.41.60.10.0 23 2374.225.729.126.212.22.10.00.00.0 24 4511.133.328.922.22.22.20.00.00.0 Total446,7410.10.96.224.540.022.45.10.70.1 In this table,the figures give the probability that the Law is off by the given amount.Thus,with 16 cards in the two suits, there is a 42.4%chance that the Law is correct,a 26.3%chance that 17 tricks can be taken by the two sides,and so on. Do these results validate or invalidate the Law Something in between

trick -- it just overestimates approximately as often as it underestimates. To evaluate the specific actions suggested by the Law, we need a bit more detail. Here it is: Error Length samples −4 −3 −2 −1 0 +1 +2 +3 +4 14 46,944 0.1 0.6 4.8 27.0 46.6 18.2 2.4 0.2 0.0 15 47,281 0.1 0.6 5.0 26.6 45.7 19.2 2.6 0.2 0.0 16 120,525 0.1 0.5 3.8 20.7 42.4 26.3 5.5 0.7 0.1 17 102,184 0.1 0.6 5.2 23.5 39.4 24.5 5.8 0.7 0.1 18 69,792 0.1 0.8 7.1 25.0 36.0 22.8 6.9 1.2 0.1 19 37,561 0.1 1.3 10.7 28.9 33.8 18.8 5.4 1.0 0.1 20 15,845 0.2 3.1 16.4 31.5 29.9 13.7 4.4 0.7 0.0 21 5,041 0.7 7.4 24.0 31.9 23.3 10.1 2.3 0.3 0.0 22 1,286 2.7 13.7 28.6 30.2 17.7 5.4 1.6 0.1 0.0 23 237 4.2 25.7 29.1 26.2 12.2 2.1 0.0 0.0 0.0 24 45 11.1 33.3 28.9 22.2 2.2 2.2 0.0 0.0 0.0 Total 446,741 0.1 0.9 6.2 24.5 40.0 22.4 5.1 0.7 0.1 In this table, the figures give the probability that the Law is off by the given amount. Thus, with 16 cards in the two suits, there is a 42.4% chance that the Law is correct, a 26.3% chance that 17 tricks can be taken by the two sides, and so on. Do these results validate or invalidate the Law ? Something in between

Clearly as the number of trumps grows,the Law becomes less and less reliable,consistently overestimating the number of tricks that can be taken by the two sides. But let's look at a lower-level,competitive situation. Suppose that both you and your opponents appear to have 9-card fits,and your opponents have bid 3.Holding spades, do you compete further? It is a consequence of the Law that you should.Assuming that the opponents have not voluntarily missed a game,9 tricks will be their limit,leaving nine for you.If 18 tricks are available,competing is correct. The table shows that 18 tricks are available 67%of the time (the Law has to be either correct or conservative in estimating the number of tricks that can be taken).So if you follow the Law,you will make the correct decision 67%of the time. For better or worse,suppose you compete to 3.The opponents,if they make the decision suggested by the Law,will not bid on if vulnerable,and probably will bid on if not vulnerable, preferring the 100 penalty to going 140 defending spades.And if you would have gone down in 3,they will actually make 4,taking 10 of the presumed 18 tricks that are available

Clearly as the number of trumps grows, the Law becomes less and less reliable, consistently overestimating the number of tricks that can be taken by the two sides. But let's look at a lower-level, competitive situation. Suppose that both you and your opponents appear to have 9-card fits, and your opponents have bid 3 . Holding spades, do you compete further? It is a consequence of the Law that you should. Assuming that the opponents have not voluntarily missed a game, 9 tricks will be their limit, leaving nine for you. If 18 tricks are available, competing is correct. The table shows that 18 tricks are available 67% of the time (the Law has to be either correct or conservative in estimating the number of tricks that can be taken). So if you follow the Law, you will make the correct decision 67% of the time. For better or worse, suppose you compete to 3 . The opponents, if they make the decision suggested by the Law, will not bid on if vulnerable, and probably will bid on if not vulnerable, preferring the 100 penalty to going 140 defending spades. And if you would have gone down in 3 , they will actually make 4 , taking 10 of the presumed 18 tricks that are available

Are these decisions right? The decision to pass 3 vulnerable is right,provided that at most 18 tricks are available to the two sides.Looking at the table,this happens 69%of the time.The decision to bid on is right if at least 18 tricks are available,just as in the previous paragraph--so that Law leads you to the right decision 67%of the time in this case. In general,the Law will lead you to do the right thing when it's either exactly right or when it's wrong in the "right"direction. Bidding on is generally right if the Law is conservative;passing is right if the Law is overestimating the number of available tricks. Either way,the probabilities are similar whenever the suit lengths combine to 20 cards or fewer,which they will 99%of the time.So here's the straight story on the Law: Provided that the trump lengths combine to 20 cards or fewer,the Law of Total Tricks will lead you to correct decisions approximately 70%of the time. Does that mean you should follow it That depends, I suppose,on whether you can make competitive judgments correctly more or less than 70%of the time without it

Are these decisions right? The decision to pass 3 vulnerable is right, provided that at most 18 tricks are available to the two sides. Looking at the table, this happens 69% of the time. The decision to bid on is right if at least 18 tricks are available, just as in the previous paragraph -- so that Law leads you to the right decision 67% of the time in this case. In general, the Law will lead you to do the right thing when it's either exactly right or when it's wrong in the "right" direction. Bidding on is generally right if the Law is conservative; passing is right if the Law is overestimating the number of available tricks.Either way, the probabilities are similar whenever the suit lengths combine to 20 cards or fewer, which they will 99% of the time. So here's the straight story on the Law: Provided that the trump lengths combine to 20 cards or fewer, the Law of Total Tricks will lead you to correct decisions approximately 70% of the time. Does that mean you should follow it ? That depends, I suppose, on whether you can make competitive judgments correctly more or less than 70% of the time without it

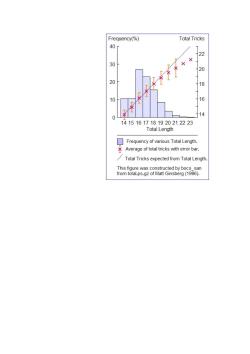
Frequency(%) Total Tricks 40r 22 + 30 20 20 18 10 16 14 0 14151617181920212223 Total Length Frequency of various Total Length. Average of total tricks with error bar. Total Tricks expected from Total Length. This figure was constructed by boco_san from total.ps.gz of Matt Ginsberg(1996)